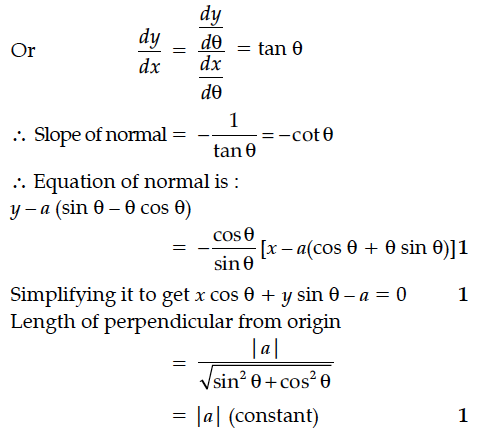
Show that the normal at any point θ to the curve x = a cos θ + aθ sin θ, y = a sin θ – aθ cos θ is at a constant distance from the origin.
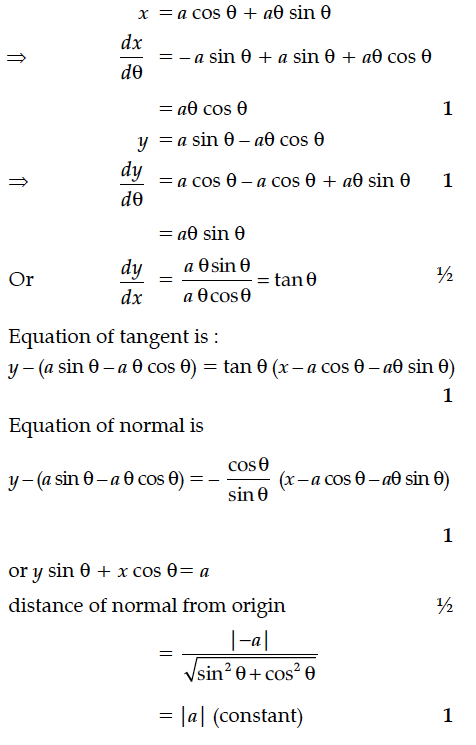
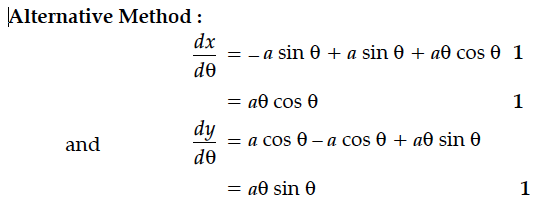
Show that the equation of normal at any point t on the curve x = 3 cos t – cos³t and y = 3 sin t – sin³t is 4(y cos2t – x sin³t) = 3 sin 4t
Find the equation of tangent to the curve y = cos (x + y), -2π ≤ x ≤ 0, that is parallel to the line x + 2y = 0.
Find the equation of tangent and normal to the curve x = 1 – cos θ, y = θ – sin θ at θ =
Find the points on the curve y = x³ at which the slope of the tangent is equal to y-coordinate of the point.
Find the slope of tangent to the curve y = 3x² – 6 at the point on it whose x-coordinate is 2.
Find the points on the curve y = x³ – 3x² – 9x + 7 at which the tangent to the curve is parallel to the x-axis.
The equation of tangent at (2, 3) on the curve y² = ax³ + b is y = 4x – 5. Find the values of a and b.
Show that the function f : R {x ∈ R – 1 < x < 1} defined by f(x) = x ∈ R is one-one and onto function. Hence find f⁻¹(x).
Consider the experiment of tossing a coin. If the coin shows head, toss is done again, but if it shows tail, then throw a die. Find the conditional probability of the events that ‘the die shows a number greater than 4’, given that ‘there is atleast one tail’.
Find the equation of the normal at a point on the curve x² = 4y which passes through the point (1, 2). Also find the equation of the corresponding tangent.
Find the angle of intersection of the curves y² = 4ax and x²= 4by.
Consider given by f(x) = Show that f is bijective. Find the inverse of f and hence find f⁻¹(0) and x such that f⁻¹(x) = 2.
Find the particular solution of the differential equation (x – sin y) dy + (tan y) dx = 0, given that y = 0 when x = 0.
Find the equation of tangent and normal to the curve x = 1 – cos θ, y = θ – sin θ at θ =
