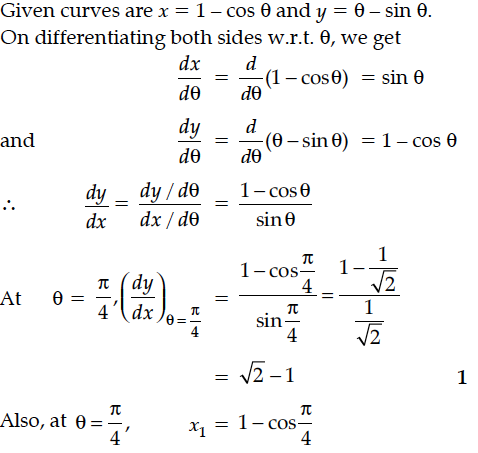
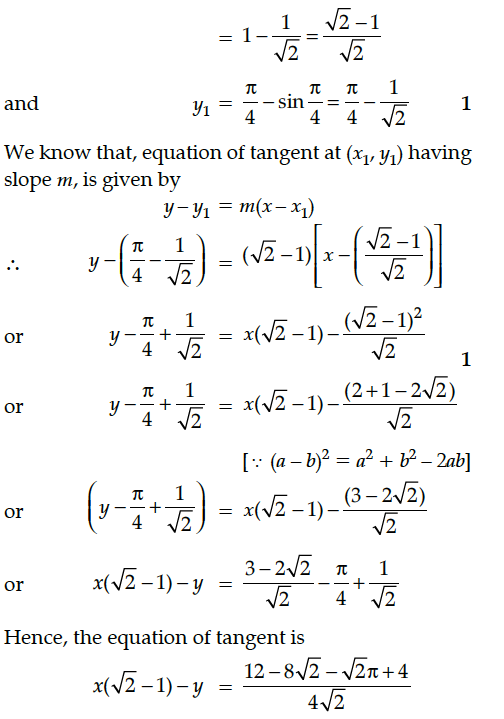
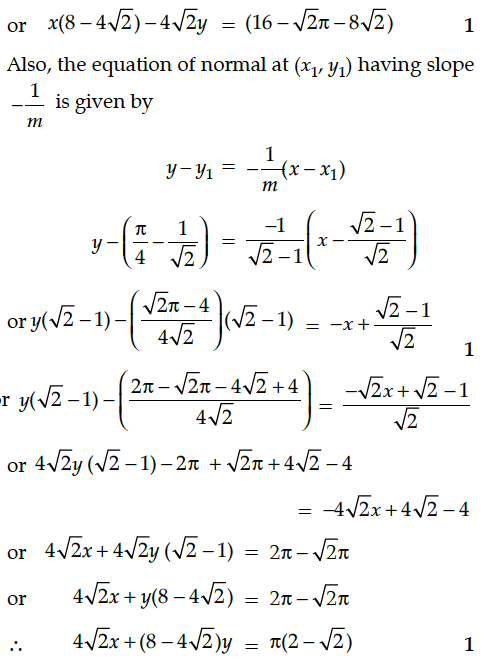
Find the equations of the tangent and normal to the curve x = a sin³θ, y = b cos³θ at
Find the equation of the tangent to the curve y = x⁴ – 6x³ + 13x² – 10x + 5 at point x = 1, y = 0.
Find the equation of tangent to the curve 4x² + 9y² = 36 at the point (3 cos θ, 2 sin ).
Find the equations of the normal to the curve y = x³+ 2x + 6, which are parallel to line x + 14y + 4 = 0.
The equation of tangent at (2, 3) on the curve y² = ax³ + b is y = 4x – 5. Find the values of a and b.
Show that the relation R in the Set A = {1, 2, 3, 4,5} given by R = {(a, b) : |a – b| is divisible by 2} is an equivalence relation. Write all the equivalence classes of R.
Find the angle of intersection of the curves y² = 4ax and x²= 4by.
Find the particular solution of the differential equation given that when x = 0, y = 0.
Find the equation of the tangent line to the curve y =x² -2x + 7 which is
(i) parallel to the line 2x-y +9 =0
(ii) perpendicular to the line 5y - 15x = 13.
Find the equation of tangent and normal to the curve x = 1 – cos θ, y = θ – sin θ at θ =
LetA = {x ∈ Z:0≤ x ≤12}. Show that R={(a,b):a,b ∈ A, |a-b| is divisible by 4} is an equivalence relation. Find the set of all elements related to 1. Also write the equivalence class [2].