If E and F be two events such that P(E) = 1/3, P(F) = 1/4, find P(E U F) if E and F are independent events.

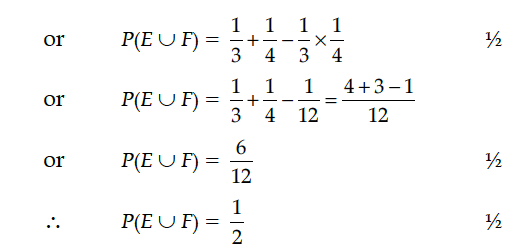
If A and B are two events such that P(A) = 0.4, P(B) = 0.8 and P(B/A) = 0.6, then find P(A/B).
If E and F are two events such that P(E) = 1/4, P(F) = 1/2 and P(E ∩ F) = 1/8, find
(i) P(E or F)
(ii) P(not E and not F).
If E and F are independent events, then show that
(i) E and F' are independent events.
(ii) E' and F are also independent events.
If A and B are two independent events such that P(A' ∩ B)= 2/15 and P(A ∩ B')= 1/6, then find P(A) and P(B).
Show that the function f(x) = x³ – 3x² + 6x – 100 is increasing on R.
Find the particular solution of the differential equation given that y(0) =
The volume of a cube is increasing at the rate of 9 cm³/sec. How fast is the surface area increasing when the length of an edge is 10 cm.
Show that the function f given by f(x) = tan⁻¹ (sin x+ cos x) is decreasing for all
