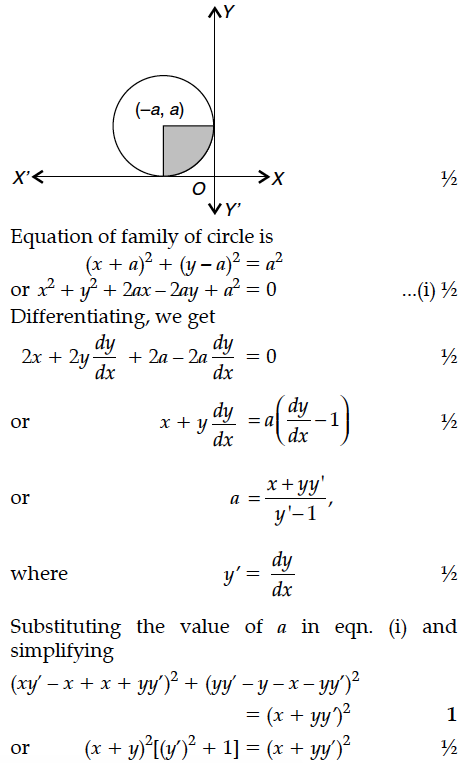
Form the differential equation of the family of circles in the second quadrant and touching the co-ordinate axes.
Obtain the differential equation of the family of circles passing through the points (a, 0) and (– a, 0).
Form the differential equation of all circles which is tough the x-axis at the origin.
From the differential equation of equation y = a cos 2x + b sin 2x, where a and b are constant.
Form the differential equation representing family of ellipses having foci on X-axis and centre at the origin.
Find the general solution of the differential equation (1 + tan y)(dx – dy) + 2xdy = 0.
Find the particular solution of the differential equation given that y = 0 when x = 1.
Find the points on the curve y = x³ – 3x² – 9x + 7 at which the tangent to the curve is parallel to the x-axis.
