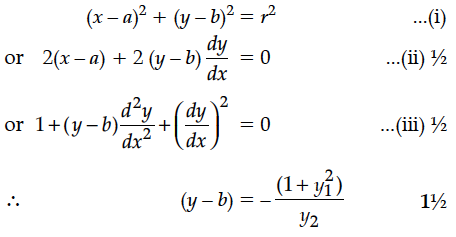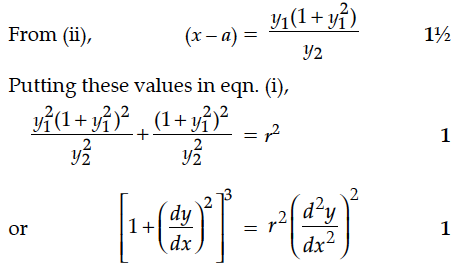Question

Let N denote the set of all natural numbers and R be the relation on N × N defined by (a, b) R (c, d) if ad(b + c) = bc(a + d). Show that R is an equivalence relation.

If A and B are two independent events such that P(A' ∩ B)= 2/15 and P(A ∩ B')= 1/6, then find P(A) and P(B).

Solve the differential equation : ${\frac{d y}{d x}}-3y\cot x=\sin2x\operatorname{given}y=2,{\mathrm{~when~}}x={\frac{\pi}{2}}.$

Find the particular solution of the differential equation $\left(1+x^{2}\right){\frac{d y}{d x}}=\left(e^{{\mathrm{mtan}^{\perp}x}}-y\right)$ given that y = 1 when x = 0.

Prove that the curves x = y² and xy = k cut at right angles if 8k² = 1.

If A = $\left(\begin{array}{l l l }1 & 0 & 2 \\ 0 & 2 & 1 \\ 2 & 0 & 3\end{array}\right)$, prove that A³ – 6A² + 7A + 2I = 0

Obtain the differential equation of all the circles of radius r.