Question

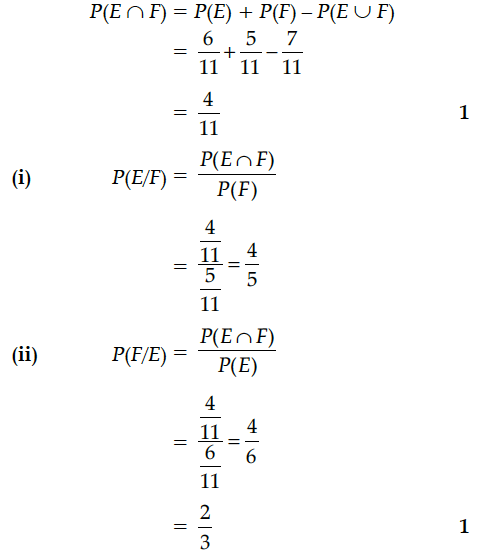
If P(E) = 6/11, P(F) = 5/11 and P(E ∪ F) = 7/11 then find (i) P(E/F) (ii) P(F/E)

The radius r of a right circular cylinder is decreasing at the rate of 3 cm/min. and its height h is increasing at the rate of 2 cm/min. When r =7 cm and h = 2 cm, find the rate of change of the volume of cylinder. $[\mathrm{Use}\:\pi=\:\frac{22}{7}\:]$

A balloon, which always remains spherical, has a variable diameter ${\frac{2}{3}}(3x+1)$ . Find the rate of change of its volume with respect to x.

If A and B are square matrices of order 3 such that |A| = –1, |B| = 3, then find the value of |2AB|.

How many equivalence relations on the set {1, 2, 3} containing (1, 2) and (2, 1) are there in all ?Justify your answer.

If A = $\begin{vmatrix}2 & 3 & -1 \\ 4 & 1 & 0 \\ 3 & 3 & 2\end{vmatrix}$ , find $M_{12}\times M_{21}+C_{21}\times C_{12}$ when $M_{i j}$ is called minor and $C_{i j}$ is called co-factors of A.

The radius r of a right circular cone is decreasing at the rate of 3 cm/minute and the height h is increasing at the rate of 2 cm/minute. When r = 9 cm and h = 6 cm, find the rate of change of its volume.