A balloon, which always remains spherical, has a variable diameter . Find the rate of change of its volume with respect to x.
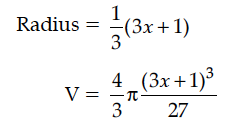
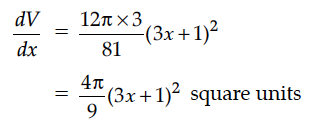
For the curve y = 5x – 2x³, if x increases at the rate of 2 units/sec, then find the rate of change of the slope of the curve when x = 3.
Using derivative, find the approximate percentage increase in the area of a circle if its radius is increased by 2%.
If the radius of sphere is measured as 9 cm with an error of 0.03 cm, then find the approximate error in calculating its surface area.
Find the slope of tangent to the curve y = 3x² – 6 at the point on it whose x-coordinate is 2.
Find the point on the curve y = x³ – 11x + 5 at which the equation of tangent is y = x – 11.
Find the equations of the normal to the curve y = x³+ 2x + 6, which are parallel to line x + 14y + 4 = 0.
The total revenue received from the sale of x units of a product is given by R(x) = 3x² + 36x + 5 in rupees. Find the marginal revenue when x = 5, where by marginal revenue we mean the rate of change of total revenue with respect to the number of items sold at an instant.
The radius r of a right circular cylinder is increasing at the rate of 5 cm/min and its height h, is decreasing at the rate of 4 cm/min. When r= 8 cm and h = 6 cm, find the rate of change of the volume of cylinder.
Find the sum of the order and the degree of the following differential equations :
From the differential equation of equation y = a cos 2x + b sin 2x, where a and b are constant.
