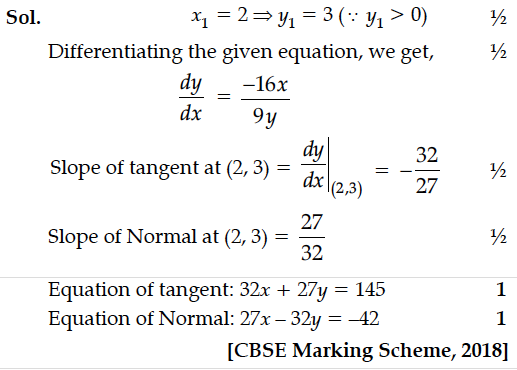
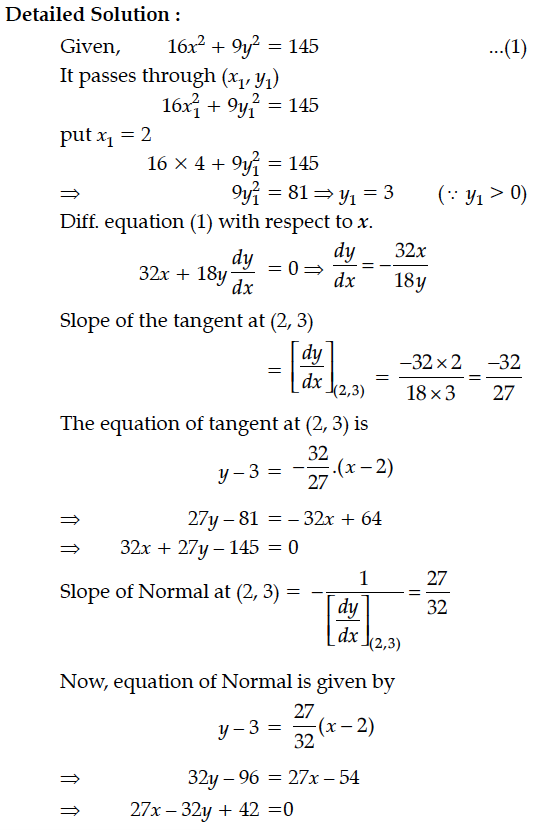
Find the equations of the tangent and the normal, to the curve 16x² + 9y² = 145 at the point where x₁ = 2 and y₁ > 0.
Find the equation of tangent to the curve 4x² + 9y² = 36 at the point (3 cos θ, 2 sin ).
Find the equations of the tangent and normal to the curve x = a sin³θ, y = b cos³θ at
Find the equation of the tangent to the curve y = x⁴ – 6x³ + 13x² – 10x + 5 at point x = 1, y = 0.
Find the equation of tangent to the curve x² + 3y = 3, which is parallel to line y – 4x + 5 = 0.
Find the equations of the normal to the curve y = 4x³ – 3x + 5 which are perpendicular to the line 9x – y + 5 = 0..
Find the equations of the normal to the curve y = x³+ 2x + 6, which are parallel to line x + 14y + 4 = 0.
Find the equations of the normal to the curve x² = 4y which passes through the point (1, 2).
Find the particular solution of the differential equation satisfying the given condition given that y = 1, when x = 0.
If the function f : R → R be defined by f(x) = 2x – 3 and g : R → R by g(x) = x³ + 5, then find fog and show that fog is invertible. Also, find (fog)⁻¹, hence find (fog)⁻¹(9).
Find the particular solution of the differential equation given that y = 1 when x = 0.
Let = , = , and = Find a vector of magnitude 6 units,which is parallel to the vector .
Form the differential equation representing family of curves given by (x – a)² + 2y²= a², where a is an arbitrary constant.
Find the general solution of the following differential equation :
For the curve y = 4x³ – 2x⁵, find all those points at which the tangent passes through the origin.
