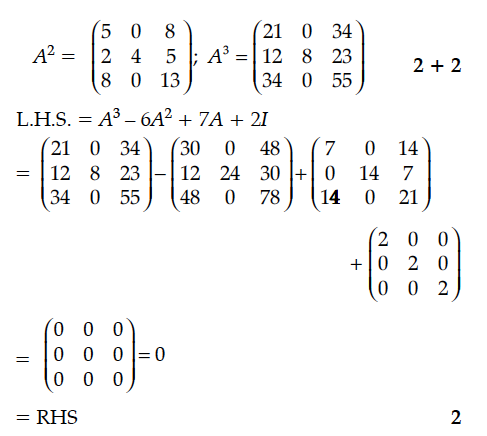
If A = , find A² – 5A + 4I and hence find a matrix X such that A² – 5A + 4I + X = 0.
Let N denote the set of all natural numbers and R be the relation on N × N defined by (a, b) R (c, d) if ad(b + c) = bc(a + d). Show that R is an equivalence relation.
Find the angle of intersection of the curves y² = 4ax and x²= 4by.
Find the equation of the tangent to the curve which is parallel to the line 4x – 2y + 5 = 0.
Find the equation of tangent and normal to the curve x = 1 – cos θ, y = θ – sin θ at θ =
Find the equations of tangents to the curve 3x² – y² = 8, which passes through the point