If P(A) = 0.4, P(B) = 0.8 and P(B|A) = 0.6, then P(A ∪ B) is equal to
(a) 0.24
(b) 0.3
(c) 0.48
(d) 0.96
(a) 0.24
(b) 0.3
(c) 0.48
(d) 0.96
Correct option : (d)
Explanation :
Here,P(A) = 0.4, P(B) = 0.8 and P(A|B) = 0.6
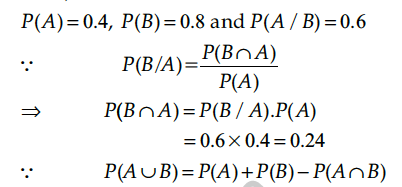
= 0.4+0.8-0.24
= 1.2=0.24 = 0.96.
Explanation :
Here,P(A) = 0.4, P(B) = 0.8 and P(A|B) = 0.6
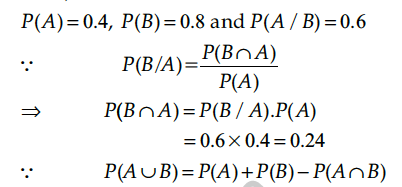
= 0.4+0.8-0.24
= 1.2=0.24 = 0.96.
If A and B are two events such that A ⊂ B and P(B) ≠ 0, then which of the following is correct?
(a) P(A|B) = P(B)/P(A)
(b) P(A|B) < P(A)
(c) P(A|B) ≥ P(A)
(d) P(A|B) ≥ P(A)
If P(A|B) > P(A), then which of the following is correct :
(a) P(B|A) < P(B)
(b) P(A ∩ B) < P(A) . P(B)
(c) P(B|A) > P(B)
(d) P(B|A) = P(B)
If P(A) = 1/2, P(B) = 0, then P(A|B) is
(a) 0
(b) 1/2
(c) not defined
(d) 1
If P(A) = 0.4, P(B) = 0.8 and P(B|A) = 0.6, then P(A ∪ B) is equal to
(a) 0.24
(b) 0.3
(c) 0.48
(d) 0.96
If A and B are any two events such that P(A) + P(B) – P(A and B) = P(A), then
(a) P(B|A) = 1
(b) P(A|B) = 1
(c) P(B|A) = 0
(d) P(B|A) = 0
If A and B are events such that P(A|B) = P(B|A), then
(a) A ⊂ B but A ≠ B
(b) A = B
(c) A ∩ B = Φ
(d) P(A) = P(B)
