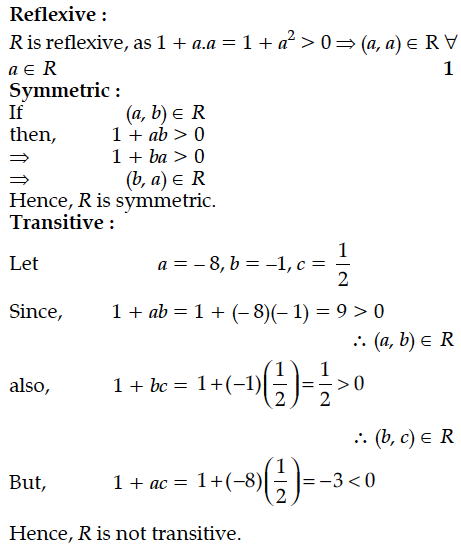
Check whether the relation R in the set R of real numbers, defined by R = {(a, b) : 1 + ab > 0}, is reflexive, symmetric or transitive.
Show that the relation S in the set R of real numbers defined as S = {(a, b) : a, b ∈ R and a ≤ b³} is neither reflexive nor symmetric nor transitive.
State the reason for the relation R in the set {1, 2, 3} given by R = {(1, 2), (2, 1)} not to be transitive.
Let R be the equivalence relation in the set A = {0, 1, 2, 3, 4, 5} given by R = {(a, b) : 2 divides (a –b)}. Write the equivalence class [0].
State the reason why the Relation R = {(a, b) : a ≤ b²} on the set R of real numbers is not reflexive.
Show that the relation R on the set Z of all integers defined by (x, y) ∈ is divisible by 3 is an equivalence relation.
Show that a function f : R → R given by f(x) =ax + b,a, b ∈ R, a ≠ 0 is a bijective.
How many equivalence relations on the set {1, 2, 3} containing (1, 2) and (2, 1) are there in all ?Justify your answer.
Solve the differential equation + given that y = 1 when x = 1.
Two farmers X and Y cultivate only three varieties of rice namely Basmati, Permal and Naura. The sale in rupees of these varieties of rice by both the farmers in the months of September and October are given by the following matrices A and B.
Find the equation of tangents to the curve y = x³ + 2x – 4 which are perpendicular to the line x + 14y – 3 = 0.
Form the differential equation representing family of curves given by (x – a)² + 2y²= a², where a is an arbitrary constant.
Find the particular solution of the following differential equation given that at x = 2, y = 1
