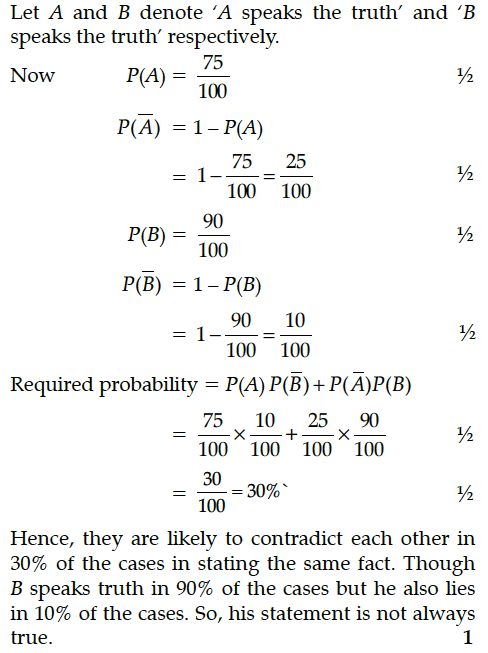
A speaks truth in 75% of the cases, while B in 90% of the cases. In what percent of cases are they likely to contradict each other in stating the same fact ? Do you think that statement of B is always true ?
P speaks truth in 70% of the cases and Q in 80% of the cases. In what percent of cases are they likely to agree
in stating the same fact ?
A speaks truth in 60% of the cases, while B in 90% of cases. In what percent of cases are they likely to contradict each other in stating the same fact ? In the cases of contradiction do you think, the statement of B will carry more weight as he speaks truth in more number of cases than A ?
A and B throw a pair of dice alternately. A wins the game if he gets a total of 9 and B wins if he gets a total of 7. If A starts the game, find the probability of winning the game by B.
A and B throw a pair of dice alternately. A wins the game if he gets a total of 7 and B wins the game if he gets a total of 10. If A starts the game, then find the probability that B wins.
A couple has 2 children. Find the probability that both are boys, if it is known that
(i) one of them is a boy
(ii) the older child is a boy.
A and B throw a pair of dice alternatively, till one of them gets a total of 10 and wins the game. Find their respective probabilities of winning, if A starts first.
A box contains 50 bolts and 50 nuts. Half of the bolts and nuts are rusted. If two items are drawn with replacement, what is the probability that either both are rusted or both are bolts.
Find the equations of tangents to the curve y = (x²– 1) (x – 2) at the points, where the curve cuts the X-axis.
To raise money for an orphanage, students of three schools A, B and C organized an exhibition in their locality, where they sold paper bags, scrap-books and pastel-sheets made by them using recycled paper at the rate of ₹ 20, ₹ 15 and ₹ 10 per unit respectively. School A sold 25 paper bags, 10 scrap-books and 30 pastel-sheets. School B sold 20 paper-bags, 15 scrap-books and 30 pastel-sheets. While school C sold 25 paper-bags, 18 scrap-books and 35 pastel-sheets. Using matrices, find the total amount raised by each school.
Find the equation of tangent to the curve y = cos (x + y), -2π ≤ x ≤ 0, that is parallel to the line x + 2y = 0.
If the radius of sphere is measured as 9 cm with an error of 0.03 cm, then find the approximate error in calculating its surface area.
Probability of solving specific problem independently by A and B are 1/2 and 1/3 respectively. If both try to solve the problem, independently, then find the probability that
(i) the problem is solved
(ii) exactly one of them solves the problem
