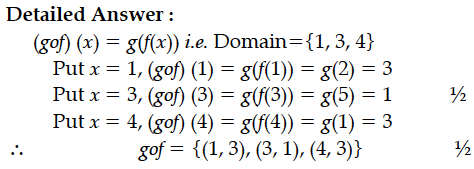
Let f : {1, 3, 4} → {1, 2, 5} and g : {1, 2, 5} → {1, 3} given by f = {(1, 2) (3, 5) (4, 1)} and g = {(1, 3), (2, 3), (5, 1)} Write down gof.
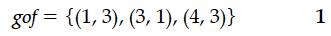
If f : R → R and g : R → R are given by f(x) = sin x and g(x) = 5x² then find gof(x)
State the reason for the relation R in the set {1, 2, 3} given by R = {(1, 2), (2, 1)} not to be transitive.
Let f : X -> Y be a function. Define a relation R on X given be R = {(a, b) : f(a) = f(b)}. Show that R is an equivalence relation ?
If A = {1, 2, 3}, B = {4, 5, 6, 7} and f = {(1, 4), (2, 5),(3, 6)} is a function from A to B. State whether f is one-one or not.
Let R be the equivalence relation in the set A = {0, 1, 2, 3, 4, 5} given by R = {(a, b) : 2 divides (a –b)}. Write the equivalence class [0].
If R = {(x, y) : x + 2y = 8} is a relation on N, writethe range of R.
