Write fog, if f : R → R and g : R → R are given by f(x) = |x| and g(x) = |5x - 2|.
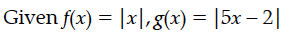
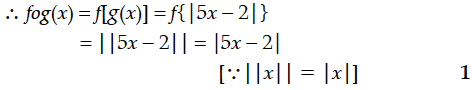
If f : R → R and g : R → R are given by f(x) = sin x and g(x) = 5x² then find gof(x)
Show that a function f : R → R given by f(x) =ax + b,a, b ∈ R, a ≠ 0 is a bijective.
If f is an invertible function, defined as f(x) = , then write
Write a unit vector in the direction of vector where and are the points (1, 3, 0) and (4, 5, 6),respectively,
If A is an invertible square matrix of order 3 and |A| = 5, then find the value of |adj A|.
Let R be the equivalence relation in the set A = {0, 1, 2, 3, 4, 5} given by R = {(a, b) : 2 divides (a –b)}. Write the equivalence class [0].
