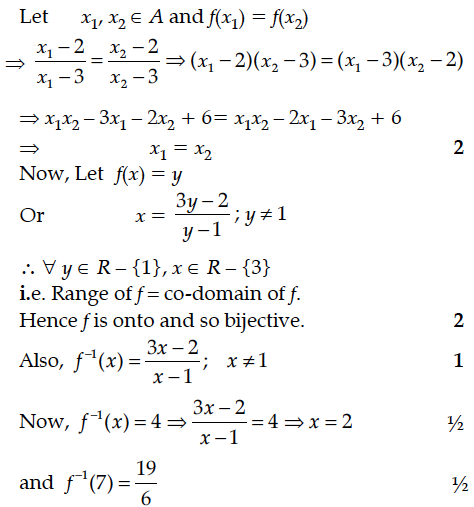
Let A = R – {2}, B = R – {1}. If f : A → B is a function defined by f(x)=(x−1x−2), show that f is one-one and onto. Hence find f−1.
Show that the function f : R {x ∈ R – 1 < x < 1} defined by f(x) =x1+|x|, x ∈ R is one-one and onto function. Hence find f⁻¹(x).
Let f : R → R be defined by f(x) = 3x² – 5 and g : R → R be defined by g(x) =xx2+1. find gof(x) .
Find the particular solution of the differential equation : dydx +ycotx= 4x cosec x, (x ≠ 0), given, that y = 0,when x=π2
Solve (1+x2)dydx+2xy−4x2=0 subject to the initial condition y(0) = 0.
Solve the following differential equation : xdydx +y−x+xycotx=0,x≠0
Using properties of determinants, solve for x :
|a+xa−xa−xa−xa+xa−xa−xa−xa+x| = 0
Check whether the relation R in the set R of real numbers, defined by R = {(a, b) : 1 + ab > 0}, is reflexive, symmetric or transitive.
Find the particular solution of the differential equation dydx =1+x+y+xy given that y = 0 when x = 1.