Find the particular solution of the following differential equation :
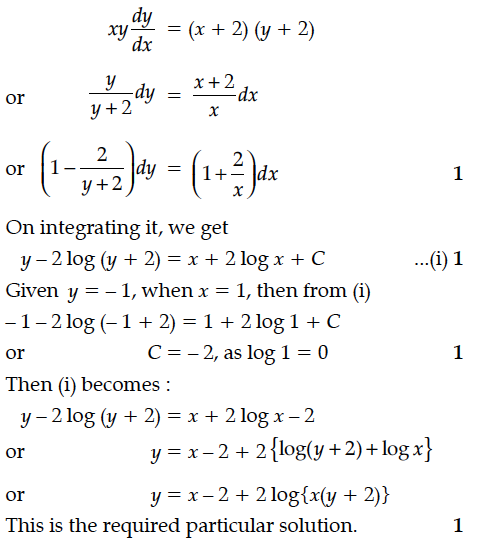
Find the particular solution of the following differential equation :
y = 0 when x = 0.
Find the particular solution of the differential equation given that y = 0 when x = 1.
Find the particular solution of the following differential equation :
given that y = 1, when x = 0.
Let f : N → N be defined as
for all n ∈ N. State whether the function f is
bijective. Justify your answer.
Prove that x² – y² = c(x² + y²)² is the general solution of the differential equation (x² – 3xy²) dx = (y³ – 3x²y) dy, where C is a parameter.
Find the particular solution of the differential equation given that y = 1, when x = 0.
P speaks truth in 70% of the cases and Q in 80% of the cases. In what percent of cases are they likely to agree
in stating the same fact ?
To raise money for an orphanage, students of three schools A, B and C organized an exhibition in their locality, where they sold paper bags, scrap-books and pastel-sheets made by them using recycled paper at the rate of ₹ 20, ₹ 15 and ₹ 10 per unit respectively. School A sold 25 paper bags, 10 scrap-books and 30 pastel-sheets. School B sold 20 paper-bags, 15 scrap-books and 30 pastel-sheets. While school C sold 25 paper-bags, 18 scrap-books and 35 pastel-sheets. Using matrices, find the total amount raised by each school.
Find the particular solution of differential equation : given that y = 1 when x = 0.
