Prove that x² – y² = c(x² + y²)² is the general solution of the differential equation (x² – 3xy²) dx = (y³ – 3x²y) dy, where C is a parameter.
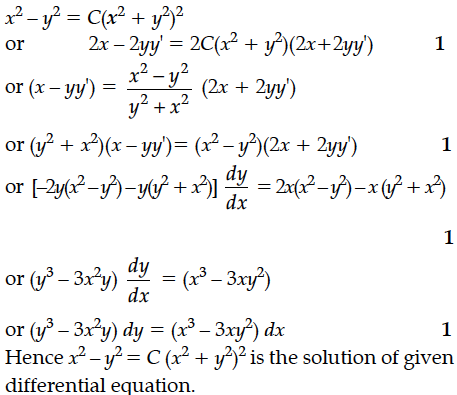
Find the general solution of the differential equation (1 + tan y)(dx – dy) + 2xdy = 0.
Find the particular solution of the differential equation (x – sin y) dy + (tan y) dx = 0, given that y = 0 when x = 0.
Find the particular solution of the differential equation x (1 + y²) dx – y (1 + x²) dy = 0, given that y = 1, when x =0.
A speaks truth in 60% of the cases, while B in 90% of cases. In what percent of cases are they likely to contradict each other in stating the same fact ? In the cases of contradiction do you think, the statement of B will carry more weight as he speaks truth in more number of cases than A ?
Form the differential equation of the family of circles in the second quadrant and touching the co-ordinate axes.
Find the general solution of the differential equation (1 + tan y)(dx – dy) + 2xdy = 0.
