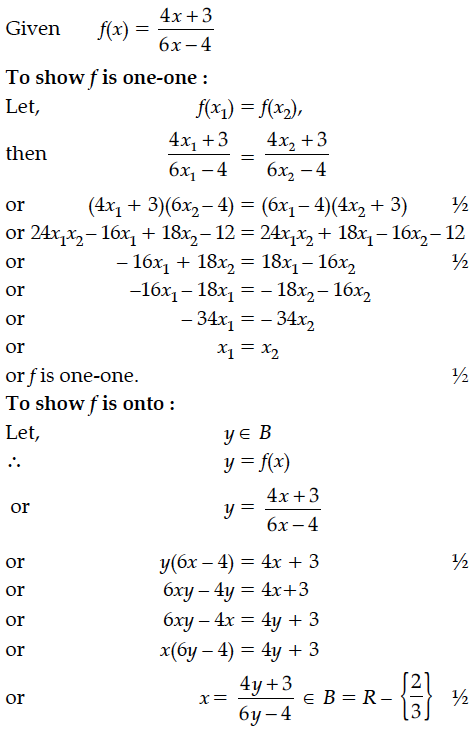
Show that the function f in A = defined as f(x) = is one-one and onto. Hence find
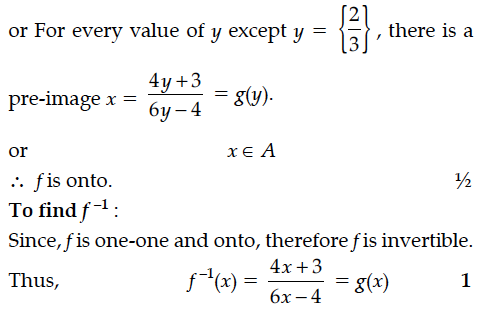
Show that the function f : R {x ∈ R – 1 < x < 1} defined by f(x) = x ∈ R is one-one and onto function. Hence find f⁻¹(x).
Show that the relation R on the set Z of all integers defined by (x, y) ∈ is divisible by 3 is an equivalence relation.
Let A = R – {2}, B = R – {1}. If f : A → B is a function defined by show that f is one-one and onto. Hence find
Find the equation of tangent to the curve at the point, where it cuts the x-axis.
Find the particular solution of the differential equation : 4x cosec x, (x ≠ 0), given, that y = 0,when
Sand is pouring from the pipe at the rate of 12 cm³/s. The falling sand forms a cone on a ground in such a way that the height of cone is always one-sixth of radius of the base. How fast is the height of sand cone increasing when the height is 4 cm ?
Find the particular solution of the following differential equation :
given that y = 1, when x = 0.
Solve the differential equation = 2 cos x, given that y = 0 when
A bag contains (2n + 1) coins. It is known that (n – 1) of these coins have a head on both sides, whereas the rest of the coins are fair. A coin is picked up at random from the bag and is tossed. If the probability that the toss results in a head is 31/42, determine the value of n.
