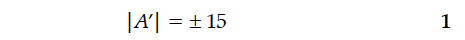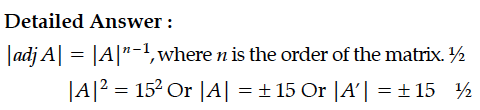Question

If A is square matrix of order 3 such that $|a d j A|=$ 64, find $|A|.$

If A is a square matrix such that A² = I, then find the simplified value of (A – I)³ + (A + I)³ – 7A.

Write the equation of tangent drawn to the curve y = sin x at the point (0, 0).

If $\begin{vmatrix} \sin\alpha & \cos\beta \\ \cos\alpha & \sin\beta \end{vmatrix} =-\ {\begin{array}{l}{1}\\ {2}\end{array}}\$ where α and β are acute angles, then write the value of α + β .

Find the differential equation representing the family of curves $V={\frac{A}{r}}+B,$ where A and B are arbitrary constants.

If the function f : R → R defined by f(x) = 3x - 4 is invertible, then find $f^{-1}.$

Write the value of the determinant : $\begin{vmatrix}102 & 18 & 36 \\ 1 & 3 & 4 \\ 17 & 3 & 6\end{vmatrix}$