If Z is the set of all integers and R is the relation on Z defined as R = {(a, b) : a, b ∈ Z and a – b is divisible by 5}. Prove that R is an equivalence relation.
The given relation is R = {(a, b) : a, b ∈ Z and a - b is
divisible by 5}.
To prove R is an equivalence relation, we have to prove R is reflexive, symmetric and transitive. Reflexive As for any x ∈ Z, we have x - x = 0, which is divisible by 5.
⇒ (x - x) is divisible by
Therefore, R is reflexive.
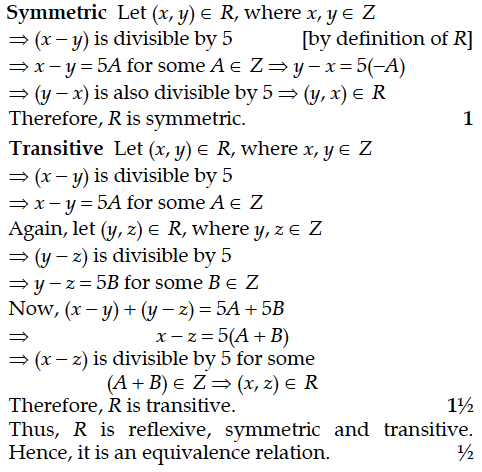
divisible by 5}.
To prove R is an equivalence relation, we have to prove R is reflexive, symmetric and transitive. Reflexive As for any x ∈ Z, we have x - x = 0, which is divisible by 5.
⇒ (x - x) is divisible by
Therefore, R is reflexive.

Let N denote the set of all natural numbers and R be the relation on N × N defined by (a, b) R (c, d) if ad(b + c) = bc(a + d). Show that R is an equivalence relation.
Let f : X -> Y be a function. Define a relation R on X given be R = {(a, b) : f(a) = f(b)}. Show that R is an equivalence relation ?
Show that the relation S in the set R of real numbers defined as S = {(a, b) : a, b ∈ R and a ≤ b³} is neither reflexive nor symmetric nor transitive.
Show that the relation R in the set N × N defined by (a, b) R (c, d) if a² + d² = b² + c² is an equivalence relation.
Show that the relation R on the set Z of all integers defined by (x, y) ∈ is divisible by 3 is an equivalence relation.
Let R be the equivalence relation in the set A = {0, 1, 2, 3, 4, 5} given by R = {(a, b) : 2 divides (a –b)}. Write the equivalence class [0].
State the reason why the Relation R = {(a, b) : a ≤ b²} on the set R of real numbers is not reflexive.
In a parliament election, a political party hired a public relations firm to promote its candidates in three ways — telephone, house calls and letters. The cost per contact (in paise) is given in matrix A as
Find the particular solution of the differential equation : x ≠ 0. Given that y = 0, when
Find the general solution of the following differential equation :
If the function f : R → R is given by f(x) = x² + 3x + 1 and g : R → R is given by g(x) = 2x – 3, then find
(i) fog (ii) gof
